WHAT IS ROCKET SCIENCE?
How do satellites work?

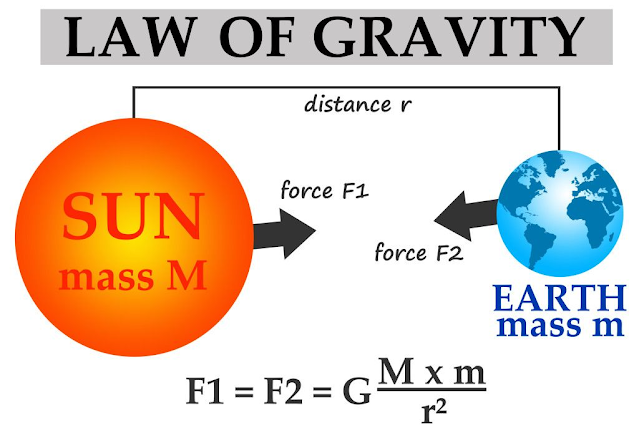
Newton’s laws of universal gravitation, published in the Principia Mathematica around 1687 also plays a role in many calculations. Keppler’s laws allow us to calculate the period and speed of such a satellite. Speed is the square root of mu over r, where mu is the standard gravitational parameter, equal to the Newtont’s gravitational constant times the mass of the planet, r is the radius of the satellite from the center of the earth. And the period has the following formula. Note that the speed and period only depends on the radius of the satellite, and not on its mass. A geo stationary orbit is circular, and since the altitude of the satellite does not change, its speed must be constant. If you do the calculations, you will find that the geostationary orbit is 35,786 km from the equator. The orbital period is 23.93 hours, or 23 hours 56 minutes. You might say, why isn’t It exactly 24 hours? Well, 23 hours and 56 minutes is actually equal to one sidereal day. This is the time it actually takes for the earth to complete one rotation with respect to a non-rotating frame of reference. The reason we normally count 24 hours as being one day, is because 24 hours is the precise time the sun is at the same spot in the sky every day. But you have to keep in mind that the earth moves with respect to the sun. The earth moves 1/365th of the arc around the sun during this time. That’s about 4 minutes. In other words, the earth has to rotate just a little bit more about 4 minutes, before the sun is directly overhead. But one full rotation around its axis is actually 4 minutes less than that.

Now the question is how is a communications satellite inserted into such an orbit? The first step in this process is to launch the satellite on a rocket that has the payload capacity to carry the satellite to this orbit, and can impart the speed necessary to maintain this orbit. In the United States, one workhorse rocket for this task has been the Atlas V. This rocket weighs about 700,000 lbs, or 317,000 kilograms at launch and can lift 28,000 lbs, or 12,700 kilograms to geostationary orbit. 90% of its weight is fuel, which is typical for rockets. The main engine is powered by liquid oxygen and RP-1 – which is a highly refined form of kerosene, similar to jet fuel. How does a rocket work? First, a rocket does not rely on the atmosphere to oxidize the fuel like a jet engine does. That’s because it carries its own oxidizer. This allows it to be able to function in outer space where there is no atmosphere available. A jet engine would not work here because there is no oxygen available to burn the fuel. Rocket engines are an application of Newton’s third law, for every action, there is an equal and opposite reaction. The combustion of fuel causes high pressure exhaust gases to be expelled at supersonic speed. The rearward acceleration of the mass of the fuel leaving the rocket nozzle causes the equal and opposite reaction of forward thrust powering the rocket forward or upward during launch. The shape of the nozzle of the rocket is designed to increase the velocity of the exhaust gases further to increase its thrust. Highest thrust is achieved when the mass flow rate of the fuel and exit velocity of the propellant is high according to this equation. The fuel has to be delivered at high volume and pressure to get the thrust required for lift. This pressure is provided by fuel pumps that boost the pressure of the gases before entering the combustion chamber. Because these pumps can boost the pressure, the fuels do not have to be pressurized so high, and the thickness of their storage tanks can be reduced resulting in weight savings, and increased payload capacity. Now you might ask, how are these pumps driven? They're typically driven by using a small amount of fuel to drive a turbine which drives the pump. Maintaining a stable straight flight is an issue. Early rockets were stabilized by large fins. For stable flight the center of pressure where the net aerodynamic force acts, must be lower than the center of gravity. This is because if its angle of attack changes relative to its flight path, the net force acting below the center gravity cam restore the stability that realigns the nose of the rocket. Modern rockets don’t use fins though, because of the extra weight and aerodynamic drag they cause. Stability comes from swiveling the thrust nozzle to keep it stable. This is called gimbaled thrust.
A geosynchronous orbit is achieved in stages. Typically, the rocket will take the satellite on its orbital altitude, but the initial orbit is elliptical. This elliptical orbit has to be changed to a circular orbit to become geostationary. So for example, An elliptical orbit may take the satellite to an altitude of 150 km, at its at its lowest point, called the perigee, and to the geo stationary orbit of 35,786 km at its highest altitude, the apogee. We can use Keppler’s laws to calculate the speeds it will have at these points – about 36,500 km/hr at perigee, and 5800 km/hr at apogee. The laws of physics are such that the satellite continues on an elliptical orbit until something changes its orbit. This change is done by accelerating the rocket at precisely the right time during its trajectory so that it forms a more and more circular orbit with every pass around the earth. The thrusters have to be turned on precisely at the apogee to accelerate the craft from 5800 km/hr to 11,000 km/hr – which is the speed it needs to have to maintain a circular geostationary orbit. As you can probably surmise, there is only one geostationary orbit and it is at 35,786 km above the earth’s equator. There is no other geostationary orbit. And there are 500 satellites at that altitude. This real estate, even in space is limited. The total perimeter available is about 265,000 km. This wouldn’t be a problem if each of the 500 satellites were placed equal distance apart, there would be 500 km of space between them. But that’s not the way the world works. There are many more satellites above the most developed regions of the earth. They are sometimes less than 10 km apart. And the speed with which they have to move is 11,000 km per hour, or 3 km per second, there is not much space.
They are less than 4 seconds apart. The real estate here has is a prized commodity, as you might imagine, and is tightly controlled by an organization called, the international telecommunications union (ITU) which assigns each satellite a slot at this perimeter. On addition, unless the rocket is launched from somewhere in the equator, it will have an orbit that is not quite geo stationary because it will not be in line or in the same plane relative to the equator. So for example, when satellites are launched from Cape Canaveral, Florida, which is located at about 28.5 degrees north latitude, the orbit will be inclined 28.5 degrees from the equator. This has to be adjusted. And this requires more fuel. It is beneficial, therefore, for countries to launch their rockets as close to the equator as possible so that less rocket fuel is needed to make this adjustment. In addition, launching from close to the equator gives the rocket added inertia because of the earth’s greater speed of spin near the equator, so that the launched rocket will already be moving at the speed of the earth's spin at the equator before the launch. Note that not all communications satellites are placed in geostationary orbits. Some are placed in low earth orbit too. Low earth orbit satellites can serve the same function, but you have to use many of them as they are moving at such high speeds. And there has to be constant hand off of transmissions from one satellite to another. But the advantage is that these satellites are cheaper to launch and cheaper to make because they don’t have to be as powerful, since transmission distances are a lot shorter.
So what happens now, that we finally have our satellite in orbit around the earth. We have adjusted to make it a circular geostationary orbit. We have placed it in a correct slot assigned by the ITU. And we have adjusted its angle of orbit so that it is in the same plane as the equator? The first thing that happens is that solar panels are deployed so that the satellite can have power to function. The main function of the satellite is to receive signals from earth mainly in the form of radio transmissions, amplify them, and relay them back at a different frequency back to the surface of the earth. The shift in frequency is used to prevent interference of incoming signals with outgoing signals. Since radio waves are a form of electromagnetic radiation, same as visible light, they do not bend much around the curvature of earth – photons are too fast after all. The job of the satellite is to transmit radio waves over long distances. Otherwise, this would require a string of thousands of relay stations on earth to do the same task. These satellites usually have at least two antennas which may be aimed at two different points on the ground. Each is used for both incoming and outgoing transmissions. These antennas are generally made as large as possible for greater sensitivity in receiving signals from earth which can become quite faint by the time they reach the satellite. But the size is limited to about 10 feet diameter, or 3 meters, due to space restrictions inside the rocket. Interestingly, a geostationary orbit is sometimes called the "Clarke orbit," named for science fiction writer Arthur C. Clarke, who wrote "2001-A space odyssey." Believe it or not, he was the first person to detail the usefulness of such an orbit in a story he wrote back in 1945. That tells you that science fiction can sometimes foretell future science fact. The next time you watch satellite TV, or use your GPS app, listen to SiriusXM radio, or check the weather, think about the rocket science and the incredible technology that goes into allowing us the privilege to enjoy these fantastical technologies.
how rockets work?
At first glance, rockets seems like almost a magical technology. The heaviest rocket ever launched was over 6 million pounds in weight, roughly as heavy as 1,500 cars, and it was launched into space. So how is such a heavy object able to rise so high to be able to break out of the earth's atmosphere? It turns out that the basic principle governing the rocket's operation is actually relatively straight forward. And that's what we want to understand today using some basic model rockets that we've built ourselves, and more importantly, that you can also build yourself. The basic principles that all rockets work by is the law of conservation of momentum, which it turns out is one of the most important ideas in all of physics. So let's quickly remember what conservation of momentum means. If we have some object like the rocket drawn on the left of the screen, which has a mass, M, and is moving with the velocity, V, then the object's momentum is defined to be the product of M and V (p=mv), the product of the mass and the velocity. And the important thing about momentum is that if we have some system that's isolated from its environment, the conservation of momentum tells us that the system's total momentum doesn't change as time goes on. That's why we say the momentum is conserved. So let's apply this to a situation, which at first glance, doesn't really seem to have anything to do with rockets, but will turn out to be quite relevant. So let's say we have a skater, who's some ice skater on some frictionless surface, who's holding a ball.

So we have the skater, which has a mass, Ms, and the ball, which has a mass, Mb. And initially, both the skater and the ball are at rest. They're moving with zero velocity. Now here, what's the total momentum of the system? Well, we add up the momentum of the skater and the ball, but since they're moving into velocity, the total momentum of the system is 0. What this tells us then, about conservation of momentum, is that at any other time which choose to look at the system, the total momentum of both the skater and the ball must be 0. But let's say that at some time the skater decides to throw the ball away from her. So we have now the ball moving at some speed, V. If we just take into account the ball, the total momentum of the system is now the mass of the ball, Mb times V, which, as we can clearly see, is not 0. So for momentum to still be conserved to make the total momentum 0, the skater must now be moving backwards with some new velocity. So the skater has new velocity, Vr or V recoil. And for that to come out to be 0 like we need by conservation for momentum, we find that V recoil is equal to f over Ms times the velocity of the ball. So let's step back and see what this actually told us. What this tells us is that if we have an object that somehow pushes away another object, the first object will get pushed right back, because momentum is conserved. Now it's easy for us to understand rockets, because they work in exactly the same way as the skater with the ball, except instead of throwing a ball, a rocket launches itself by injecting some of its own mass downward. In fact, while a rocket is being accelerated upwards, its engine is continuously all the time ejecting some mass and forcing it downwards. Because this mass is forced downwards by conservation of momentum, the rocket must then be forced upwards. But in terms of forces, the rocket has to exert some force on the ejected mass to push it downwards, which then results in an equal and opposite reaction force pushing the rocket upwards. And that's it. That's how conservation of momentum, or equivalently, Newton's third law underlies the launching of rockets.
How does that actually happen in a model rocket? It all occurs in this device shown here, which is the engine of our model rocket. If we could see the inside of the engine, it would look something like this. There are three different kinds of propellants, which are all held inside the engine by some clay, which has an opening only on the downward facing side. And what we do when we launch the rocket is we put an igniter in the engine. It was just a piece of wire with the material that catches fire when we pass a current through, which we do by hooking it up to a battery. So when the igniter catches fire, it ignites a propellant which quickly turns from a solid into a hot gas, which bounces all around the engine and then escapes the only way it can-- downwards. This results in a continuous injection of fuel downwards, which is what propels the rocket upwards. And that's it. Now that we understand the theory of how a rocket works, let's take a look at a real rocket launch in action.Rocket science is a primary branch of aerospace engineering. Thanks for reading my full article. Like this article? Follow me for more on rocket science and robotics.
Thanks for reading: How Rockets work || ROCKET SCIENCE explained And How do satellites work? , Sorry, my English is bad:)